A mathematical simulation study reveals the secrets to bowling strikes

Bowling, a sport in which players roll a ball to knock down 10 pins, is a popular sport in the United States, with over 45 million participants, and large amounts of prize money are offered at tournaments held in the United States every year. A research paper has been published by a joint research team from Loughborough University, Massachusetts Institute of Technology, and other institutions that uses mathematics to explain bowling.
Using physics simulations to find targeting strategies in competitive tenpin bowling | AIP Advances | AIP Publishing
The physics of bowling strike after strike - Ars Technica
https://arstechnica.com/science/2025/04/the-physics-of-bowling-strike-after-strike/
One of the research team, Curtis Hooper of the Loughborough University Institute of Sport Technology, is a former coach of the England team at the European Youth Championships and has experience as a competitive bowling coach. Hooper has also published several papers on bowling, including an analysis of the British national tournament and an analysis of the conditioner (oil) used on bowling lanes.
In this study, six simultaneous differential equations using Euler's equations of motion were derived to describe the motion of a bowling ball. Euler's equations of motion are equations derived by the 18th century Swiss mathematician Leonhard Euler and are used to describe the rotational motion of a rigid body. In this study, the bowling ball is modeled as a 'rigid body with an asymmetric weight block inside.'
The derived simultaneous differential equations describe the motion of the ball. The equations consist of the ball's velocity (ν), the ball's angular velocity (ω), the coefficient of friction between the ball and the lane (μ), the acceleration due to gravity (g), the ball's mass (m), the ball's principal moments of inertia (I'), and the orientation of the weight blocks on the ball (φ).
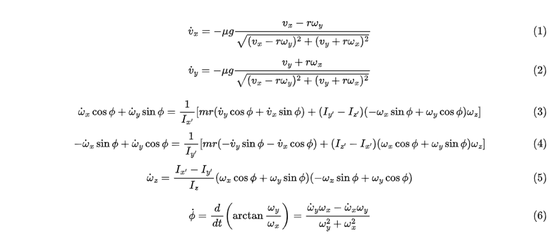
The research team then simulated all combinations of throwing positions and angles based on this simultaneous differential equation. Using data published by the United States Bowling Association in 2009 (PDF file) , the ideal strike standard was set as 'the ball hits a point 6 cm away from the center of the head pin at about 6 degrees,' and the team calculated what throwing conditions would maximize the probability of a strike. Bowling lanes are oiled, but in this simulation, a 'flat oil pattern' in which oil is evenly applied over a 40-foot (about 12.2 m) length and a 'short oil pattern' in which oil is unevenly applied over a 35-foot (about 10.7 m) length are assumed.

As a result of the simulation, it was found that the strike rate of the flat oil pattern was up to about 75% at the 20th board pitch position and 3.0 degree pitch angle, and the strike rate of the short oil pattern was up to about 89% at the 28th board pitch position and 1.8 degree pitch angle. The research team noted that the strike rate of the short oil pattern was clearly higher.
To explain this difference, the team took into account the reality that even the best professional bowlers make an error of about 0.1 degrees from their intended throw angle, which can result in a deviation of several centimeters at the back of the lane, and modeled human error as a normal distribution , then introduced the concept of 'mistake room.' This mistake room is the tolerance range in which a bowler can deviate slightly from their intended target and still have the ball reach the ideal pin contact position.
The area around the 28 board of the short oil pattern is the boundary between the low friction area in the center of the lane and the high friction area at the edge of the lane, and even if the throwing position is slightly off, it tends to be corrected naturally. The research team claims that playing on the boundary where the change in friction is large maximizes the room for error and increases the strike rate.

This assertion is consistent with the intuitive understanding of experienced bowlers. Professional bowlers empirically aim for routes that attack the friction boundary, and the research team argued that this simulation proves that this strategy is physically and mathematically correct. The research team also suggests further improvements to the model as future prospects, planning research to incorporate more detailed factors such as the effects of uneven lane topography, contact on surfaces that take into account the hardness of the ball, and the effects of ball speed and axis rotation on miss room. Future work also includes experimental measurement of more accurate friction coefficients for specific bowling ball surfaces, oil types, and lane surfaces.
Related Posts:
in Science, Posted by log1i_yk